Boston Red Sox pitcher Josh Beckett put together an amazing postseason performance this year to help the Sox to a World Series victory. He won Game 1 of the Division Series against the LA of Anaheim Angels with a 4-hit shutout. He took MVP honors in the American League Championship Series, winning Game 1 and Game 5 against the Cleveland Indians. He then topped it all off with a Game 1 victory against the streaking Colorado Rockies, who fell to the Red Sox in a four-game sweep. Beckett’s outstanding postseason was merely the cherry on top of a fine regular season which put him in contention for the AL Cy Young Award.
I decided to take a look at Josh Beckett through the lens of PITCHf/x for several reasons. One, he is an outstanding pitcher and as such is interesting to analyze. Two, he’s known as basically a two-pitch pitcher: fastball, curveball. Granted, they must be two very good pitches, but that’s unusual for a top-flight starting pitcher. Three, I was inspired by some of the discussion between Eric Van and Alan Nathan at the Sons of Sam Horn message board after his dominant Division Series shutout. Van and Nathan identified three types of fastballs among Beckett’s pitches in that game, and I was curious if I could or would see the same thing. Finally, I wanted to try out a few new ideas regarding pitch classification, and Beckett seemed like as good a vehicle as any.
First, the scouting report on Beckett. As I mentioned earlier, Beckett is basically a two-pitch pitcher. He has a mid-nineties fastball and an excellent curveball. He also mixes in a changeup and supposedly has a slider. To quote Dugout Central, “Beckett hasn’t used his slider much in the second half. It’s an inconsistent pitch with average break to it.” In the 1530 pitches recorded by the PITCHf/x system, about half the pitches Beckett threw this year and weighted more heavily toward the second half, I find no evidence that Beckett threw even a single slider.
Without further ado, I’ll present my pitch speed versus spin direction graph showing Beckett’s pitch types. (For more detail about how I derive those parameters, read my article about Jonathan Papelbon.) Then, I’ll discuss how I arrived at the pitch classification shown. Pitch speed is in mph, measured 50 feet from the plate, and spin direction is the direction of the spin axis of the pitch in degrees, as seen by the batter and catcher, with the direction of corresponding spin-induced movement to a right-handed batter noted at the bottom of the graph.
Beckett’s curveball is easy to identify. It’s a classic curveball, running 75-80 mph. I won’t spend much more time on the curveball, since for pitch classification purposes it’s typically the easiest pitch to identify, and Beckett’s is no exception. I will note the absence of sliders on the graph. The closest Beckett comes is a few slurvy curveballs, but given their slow speed and sub-110-degree spin direction, I don’t see any reason to try to split them off as a separate pitch type. They move like normal curveballs from any other pitcher.
Separating the changeups from the fastballs is where it starts to get a little interesting. Beckett doesn’t throw many of them, less than 10% of our sample, totaling 135 pitches. Speed-wise, his changeup shows quite a bit of variation, ranging from 83-93 mph. Clearly the slower pitches are changeups, but as we get up around 90 mph and into the low nineties, how can we tell the changeups from the fastballs? In Beckett’s case, it’s quite helpful to look at the data on a start-by-start basis. Almost every start has a clear separation of pitches into three groups by speed, with the changeups in the middle group.
I’ve highlighted the middle speed group in red. The x-axis in this graph is pitch sequence recorded by PITCHf/x throughout the season, which roughly corresponds to time, such that each of Beckett’s starts is compressed into a vertical line of the pitches that were recorded at various speeds. With the exception of a few pitches, it’s quite easy to separate the fastballs, changeups, and curveballs using this graph alone.
On a side note, I don’t know the reason for the almost 6-mph variability in Beckett’s top fastball speed from start to start. Perhaps an avenue for further investigation, possibly already covered by Joe P. Sheehan’s article or Josh Kalk‘s error correction, in any case, an avenue I will bypass for now.
If you adjust the pitch speeds by normalizing the average fastball speed for each start to the overall average fastball speed and adjusting other pitches from that start by the same amount, you get the following pitch speed vs. spin direction graph:
You can see that within a given start, Beckett’s fastball speed is very consistent in the (normalized) 93-97 mph range. This is true for all three types of fastballs, which is quite unusual. Typically, a pitcher’s two-seam fastball will be a few miles per hour slower than his four-seamer, and a cutter may be even a few mph slower than that. For those pitchers, pitch speed can be an important clue in determining pitch type. That’s one reason that the pitch speed vs. spin direction graph is such a favorite of mine.
For Beckett, however, pitch speed is nearly useless in determining what types of fastballs he throws. In Van and Nathan’s Sons of Sam Horn discussion, they identify three types of fastballs: a four-seamer, a two-seamer, and a cut fastball, and in that October 3rd start the three types are fairly readily identifiable. In many of his other starts, however, the differences among them are not as obvious.
We can see a hint of the three groupings in the normalized pitch speed vs. spin direction graph, but it’s far from clear. The spin rate vs. spin direction graph proves to be a little more helpful. Spin rate is shown in revolutions per minute (rpm).
In order to see what’s going on with the fastballs, let’s zoom in on that section of the graph.
The divisions between the fastball groupings are somewhat arbitrary, but I believe they are generally well representative of reality. There is a dense cluster of pitches in the middle of the graph between 210-220 degrees, which is an appropriate spin direction for a four-seam fastball from a pitcher with a 1 o’clock delivery. Two-seam fastballs should have a greater spin direction, reflecting the sidespin commonly applied to the ball using the two-seam grip. The cluster of pitches around 230-240 degrees appear to be two-seamers. Finally, there is a small tail of pitches with spin direction of less than 200 degrees and slightly lower spin rates. These appear to be the cut fastballs. Some of them can clearly be identified as cutters if you look at the pitches on a start-by-start basis. You might argue a little with the exact delineation between pitch types here, but I think I’ve nailed it pretty closely. I can’t imagine try to hit a 95-mph fastball from Beckett while trying to decide whether it would hop, sink, or cut.
Finally, I wanted to add in a couple novelties and solicit your feedback on whether these new methods of presenting the data are helpful. These ideas mainly have their genesis in various discussions about the PITCHf/x data presentation topic at Tom Tango and Mitchel Lichtman’s The Book blog.
First, here is a graph of pitch time vs. spin direction. The horizontal axis is the same as for most of the graphs above. The vertical axis shows the time, in seconds, for the pitch to travel from shortly after the pitcher’s release point until the ball crosses the front of home plate. (In the PITCHf/x coordinate system, this is from the initial measurement point at y=50 feet to the final measurement point at the front of home plate at y=1.417 feet. The origin of the coordinate system is at the point of home plate, and the plate is 17 inches, or 1.417 feet, deep.) You could make a graph showing the time for the pitch to travel any other distance you wanted to see. The main point of this graph is to illustrate what the pitches look like on a time scale rather than the more familiar speed scale. Just over a third of a second to hit a Beckett fastball–wow!
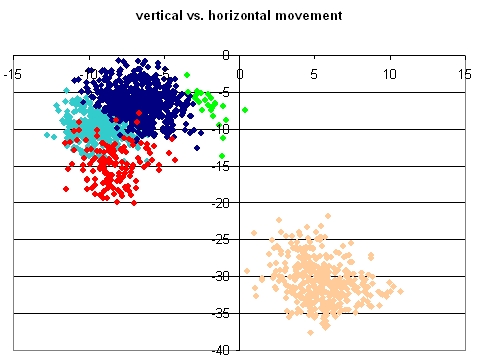
Next, I revisit the traditional PITCHf/x vertical and horizontal “break” parameters, pfx_x and pfx_z, or as Tom Tango has at least temporarily convinced me to call them, the horizontal and vertical spin movement. This graph shows the spin-induced movement of the pitches, in inches, between the y=40 feet point and the front of the plate, from the perspective of the batter/catcher.
For comparison, take a look at Josh Kalk’s algorithmically generated player card for Josh Beckett. He has a lot of good data there that you may find interesting, although he does not separate Beckett’s fastballs by type. But the main point of the preceding graph was to contrast with a graph which Tango and MGL have asked for, the spin plus gravity movement, i.e., the deviation of the pitch from a straight line, which I present below.
This graph includes all the information of the traditional pfx_z vs. pfx_x graph, but it also shows that slower pitches drop more because the force of gravity has longer to act on them. Some of Beckett’s curveballs break down almost three feet when the effect of gravity is included.
That’s all for now. I hope to take a further look at some of this data when I get a chance.
Here’s a link to Part 2 of my Josh Beckett analysis.







November 6, 2007 at 2:55 pm
Nice analysis, Mike.
November 7, 2007 at 9:37 pm
Fanstastic stuff Mike. I posted a link to my site (click name), with a tiny suggestion for the orientation. Otherwise, great stuff.